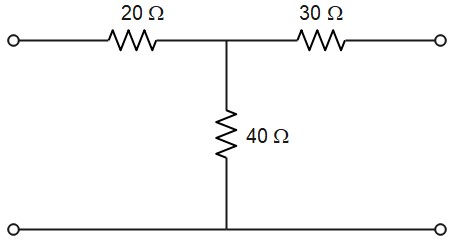
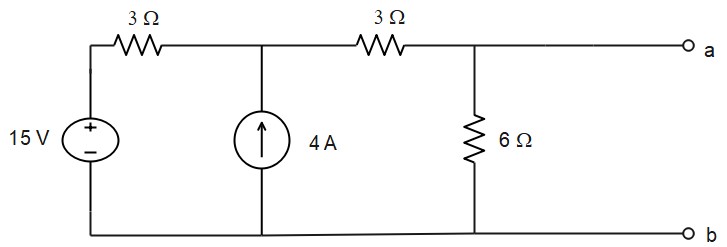
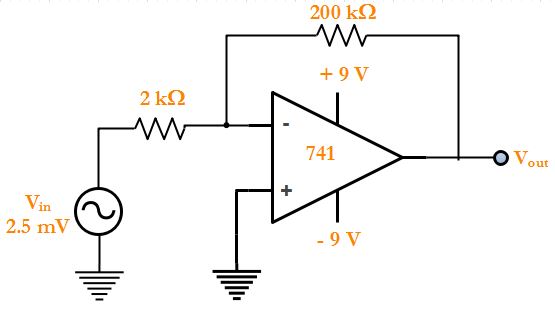
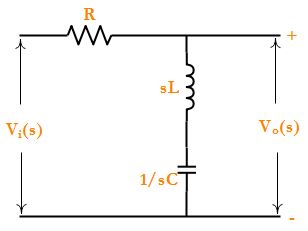
Hello Users
Our qualexe service will not be available in between 11:30 pm to 4:00 am due to server maintenance activity
 GSECL
GSECL